Is the iReady Math Test Hard?

iReady Math Test Explained: Sample Questions (2024)

Hi, I'm Liron. iReady Expert at TestPrep-Online
Have a question? Contact me at:

The iReady Math test is an adaptive assessment designed to evaluate and enhance a student's math skills. Along with the i-Ready Reading it's a crucial component of the iReady educational software by Curriculum Associates, used in many schools across the United States.

Key features of the iReady Math test include:

Adaptive testing: Questions adjust in difficulty based on student responses
Comprehensive coverage: Assesses various math domains from K-8
Detailed reporting: Provides insights into student strengths and areas for improvement
No strict time limit: Students can take their time to complete the test

🚀 Ready to ace your iReady Math test?

Get our comprehensive iReady Math Prep Pack now and boost your scores!

iReady Math Sample Questions

The iReady Math test is designed to evaluate students' proficiency in a wide range of math subtopics, including number and operations, geometry, data and statistics, algebra, measurement, problem solving, and mathematical reasoning. Below, you will find a selection of i-Ready sample questions that reflect the diverse content and formats that appear in the I-Ready Math test:

Numbers and Operations

Questions can include any math problem that asks you to apply basic operations, such as addition, subtraction, multiplication, and division, in order to reach the answer.

Question 1:

Sharon drove for two hours at 30 miles per hour and then for one hour at 60 miles per hour. What was Sharon's average speed for the journey?

A. 40 mph

B. 45 mph

C. 47.5 mph

D. 50 mph

E. 55 mph

View Explanation

The correct answer is (A).

One method to solve this is by using the formula for average speed:
Average Speed = ^{Total Distance}⁄ _{Total Time}
To find the total distance she traveled, add up the distances from each part of her journey:

First part: 30 mph for 2 hours = 30 x 2 = 60 miles.
Second part: 60 mph for 1 hour = 60 x 1 = 60 miles.
Total distance = 60 + 60 = 120 miles.

The total time she traveled was 2 hours + 1 hour = 3 hours.

Therefore, average speed = 120 miles/3 hours = 40 mph.

Another method is to use the two speeds given in the question. Sharon spent 2/3 of her journey going at 30 mph and only 1/3 going at 60 mph. So, find 2/3 of 30 and add it to 1/3 of 60:

2/3 of 30 = 30 ÷ 3 × 2 = 20.
1/3 of 60 = 60 ÷ 3 = 20.

Therefore, average speed = 20 + 20 = 40 mph.

Student tip

When dealing with average speed problems, always remember the fundamental formula: Average Speed = Total Distance ÷ Total Time. Break down the journey into segments, calculate the distance for each segment, and then sum them up. This approach helps you organize the information and solve the problem step-by-step, reducing the chance of errors.

📊 Want More Practice Questions Like These?

Our iReady Math Prep Packs includes hundreds of similar questions to boost your confidence.

Algebra extends these operations to work with variables and more abstract mathematical structures, allowing for the representation and solution of a wide range of mathematical problems. Let’s look at a question that requires algebraic thinking.

These questions ask you to solve problems or equations when there is at least one unknown variable, often represented by a letter, and you may need to find the value of that variable or else use the values you do know to determine potential outcomes.

Question 2:

Every day a factory makes 25 more T-shirts than pants. Complete the table, using the equation

t = 25 + p

A. 160

B. 165

C. 170

D. 175

View Explanation

The correct answer is (B).

In this question, the letter t stands for the number of T-shirts made and the letter p stands for the number of pairs of pants made.
The equation t = 25 + p means that to find t, you need to add 25 to p.

If p is 140, then to find t, you must add 25:
140 + 25 = 165.

Student tip

In algebraic equations, pay close attention to the relationship between variables. In this case, t = 25 + p shows that t is always 25 more than p. When filling in tables or solving similar problems, use this relationship consistently. Practice translating word problems into equations to strengthen your algebraic thinking skills.

The Measurement and Data section is a unique and important part of math assessments, testing practical skills and real-world applications of mathematical concepts.

These questions may ask you to analyse information in a chart or calculate values based on the data provided, or you may be asked to convert or calculate measurements in varying systems of measurement.

Question 3:

The number of lectures given during the week in the community center of Sedona, AZ:

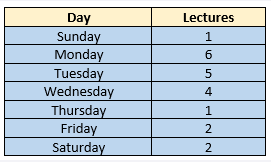
What is the range of the lectures given during the week?

A. 1

B. 2

C. 4

D. 5

E. 6

View Explanation

The correct answer is (D).

Range is the difference between the greatest number and the lowest number in a set of numbers.

First, find the greatest number in this set. The greatest number is 6 (six lectures are given on Monday).

Next, find the lowest number in this set. The lowest number is 1 (one lecture is given on Sunday and Thursday).

Now, subtract the lowest number from the greatest number:
6 – 1 = 5

The range of this set is 5.

Student tip

When working with data sets, especially in charts or graphs, familiarize yourself with basic statistical terms like range, mean, median, and mode. For range problems, quickly identify the highest and lowest values in the data set. This skill will help you efficiently analyze data in various formats.

Geometry questions on math tests are unique in their emphasis on visual-spatial reasoning, application of specific rules and formulas, and real-world relevance, while also testing logical thinking and problem-solving skills in ways distinct from other areas of mathematics.

These questions ask about the properties and dimensions of shapes, including lines and angles, and may also involve calculating missing or unknown values, such as area.

Question 4

Use the rectangle to answer the question.

What will be the effect on the area if the side length, 6, is doubled?

A. The area will stay the same.

B. The area will halve.

C. The area will double.

D. The area will be squared.

E. The area will be multiplied by six.

View Explanation

The correct answer is (C).

One option is to consider the area of the rectangle before and after and see what the change was.

The current area is length x width = 6 x 2.5 = 15. Split up 2.5 into 2 + 0.5 to calculate:

6(2 + 0.5) = 12 + 3 = 15

If the side length of 6 is doubled, then the dimension will be 12, as 6 x 2 = 12.

Now, find the area:

Area = l x w = 12 x 2.5 = 30.

Calculate in the same way:

12(2 + 0.5) = 24 + 6 = 30

The new area, 30 is double 15, the previous area.

Another option is to think about it logically. Currently the area is l x w = 2.5 x 6.
If the side length is doubled, then the new area will be 2 x l x w = 2 x 2.5 x 6

The second expression is twice as big as the first one, therefore the area will be doubled.

Student tip

In geometry problems involving area changes, think about how altering one dimension affects the overall area. Remember that area is calculated by multiplying length by width. If you double one side, you're essentially multiplying the original area by 2. Visualizing these changes can help you understand the relationships between dimensions and area more intuitively.

🎯 Maximize your preparation with our iReady Math Prep Packs.

It includes full-length simulations, targeted practice, and expert tips. Order now and start improving!

I-Ready Classroom Mathematics is an educational program and is an integral part of the I-Ready educational platform, working hand in hand with the I-Ready Math test to create a comprehensive learning experience.

When students take the I-Ready Math test, it serves as an assessment tool, gauging their math skills and adaptively identifying their strengths and weaknesses. The results of this assessment form the foundation for personalized instruction within I-Ready Classroom Mathematics.

This instructional program is designed to support educators in the classroom. It provides a structured curriculum with lessons, activities, and practice exercises that are specifically tailored to align with each student's individual needs and learning objectives, all based on their performance in the i-Ready Math test.

By leveraging the data from the I-Ready Math test, I-Ready Classroom Mathematics offers targeted instruction, allowing students to focus on areas where improvement is needed.

The iReady Math diagnostic is a crucial part of the iReady system. It:

Assesses student skills across multiple math domains
Adapts to each student's level
Provides detailed performance data
Informs personalized instruction plans

This diagnostic forms the foundation for targeted learning within the iReady Classroom Mathematics program, ensuring each student receives tailored support as understood by their iReady Math scores.

The iReady Math Test is structured to assess students across various grade levels, from Kindergarten through eighth grade. Each level corresponds to specific mathematical skills and concepts appropriate for that grade. Here’s a breakdown of the i-Ready Math grade levels and the focus areas for each:

i-Ready Math Grade Levels and Focus Areas

Level AA – Kindergarten: Focuses on foundational skills such as number recognition, counting, and basic shape identification.
Level A – First Grade: Assesses number sense, basic addition and subtraction (up to 20), simple fractions, and problem-solving skills.
Level B – Second Grade: Covers addition and subtraction (up to 100), basic multiplication concepts, and measurement skills.
Level C – Third Grade: Includes multiplication and division (up to 100), fractions, and concepts of area.
Level D – Fourth Grade: Focuses on fractions, decimals, and multi-digit multiplication.
Level E – Fifth Grade: Assesses division of larger numbers, operations with fractions, and an introduction to volume concepts.
Level F – Sixth Grade: Covers advanced arithmetic, introductory algebra, and basic geometry.
Level G – Seventh Grade: Focuses on algebraic expressions, ratios, and probability.
Level H – Eighth Grade: Includes linear equations, functions, and more complex geometry concepts.

The program's levels are linked to the i-Ready Math Diagnostic Test, which assesses a student's proficiency in various math skills and places them at an appropriate level. The Classroom Mathematics program provides lessons and materials according to the student’s level on the test, ensuring that instruction is tailored to each student's needs. This approach facilitates a personalized and adaptive learning experience, catering to the unique strengths and challenges of each student in mathematics.

Prepare Your Child for iReady Kindergarten Success

Get the iReady Kindergarten Math Practice Pack today and give your child the tools they need to excel on the adaptive iReady assessment. Expose them to a wide range of math concepts and difficulty levels, helping them master the foundational skills they'll need to thrive.

A "good" score on the iReady Math Diagnostic test depends on the grade level of the student and their individual learning goals. Generally, a good iReady math score that is above the 90th percentile is considered excellent.

A good score would typically be:

At Grade Level
One Grade Above
Two Grades Above
Three Grades Above

For example:

For a 4th grader, a score of 451-571 would be considered "At Grade Level", while 572 and above would be above grade level.
For a 7th grader, 593-620 is "At Grade Level", with 621 and above being above grade level.

Calculation of i-Ready Math Scores

Overall Scale Score: The scores range from 100 to 800, reflecting the student's overall performance. (This is similar to the iReady Reading Score but the range is different).This score is derived from the aggregate of all questions seen by the student, factoring in both the accuracy and difficulty level of the questions attempted.

Domain-Level Scores: In addition to the overall score, the test provides domain-specific scores that highlight a student's strengths and areas for improvement in specific math topics.

Norms and Percentiles: The scores are compared against national norms to determine percentile rankings. This comparison shows how a student's performance measures up against peers in the same grade, helping to identify whether they are performing at, above, or below grade level

iReady Math Test Prep Pack

Unlock your child's potential in Math.

This comprehensive resource provides targeted practice that aligns perfectly with the i-Ready curriculum.

Purchase the iReady Math Test Prep Pack today and watch their math skills soar

Preparing for the i-Ready Math test requires a strategic approach tailored to its adaptive format and content. Here are some exclusive tips to help you or your child excel on the i-Ready Math test:

Understand the Adaptive Nature: Recognize that the i-Ready Math test adapts to your performance. If you answer questions correctly, they will become more challenging. Conversely, if you struggle with certain concepts, the questions will become more focused on those areas and less complex. Embrace the adaptability as it tailors the test to your specific needs.
Use i-Ready Classroom Mathematics: If you have access to the i-Ready Classroom Mathematics program, use it to your advantage. This program offers lessons and practice exercises tailored to your skill level, as determined by the diagnostic assessment. It's an excellent resource for targeted practice.
Practice with Full-Length Simulations: To get a feel for the test's timing and format, consider practicing with full-length simulations. These simulations mimic the actual test, allowing you to experience the adaptive nature and question variety firsthand. This can help you build endurance and reduce test-day stress, as you'll be more familiar with what to expect.
Focus on Foundational Skills: Pay extra attention to foundational math skills that serve as building blocks for more advanced concepts. Mastering topics such as addition, subtraction, multiplication, division, fractions, and basic geometry will provide a strong base for tackling higher-level math questions.

Remember that the i-Ready Math test is designed to support your learning journey by identifying areas for improvement and offering personalized instruction. Embrace the process, stay motivated, and use these tips to excel on the test.

The highest level in i-Ready math is Level H (8th Grade), offering a comprehensive exploration of advanced mathematical concepts. Students at this level delve into topics such as exponents, linear equations, systems of equations, functions, and geometric transformations.

In Level H, students also analyse scatter plots and gain a solid foundation for tackling high school-level mathematics. Level H equips students with the skills and knowledge needed to handle more complex math challenges.

i-Ready Math assessments typically do not allow the use of external calculators. The assessments are designed to evaluate students' math skills, understanding, and problem-solving abilities without the assistance of calculators or other external tools.

The difficulty of the i-Ready Math test varies depending on your grade level and your math skills. It adapts to your abilities, so it can be challenging if you're strong in math or easier if you need more help.

Your level of preparation and familiarity with math concepts also plays a significant role in how difficult it feels. In general, it's designed to assess and help you improve your math skills at your grade level.

iReady Math

What is the iReady Math Test?